ma521: Representation Theory - w25
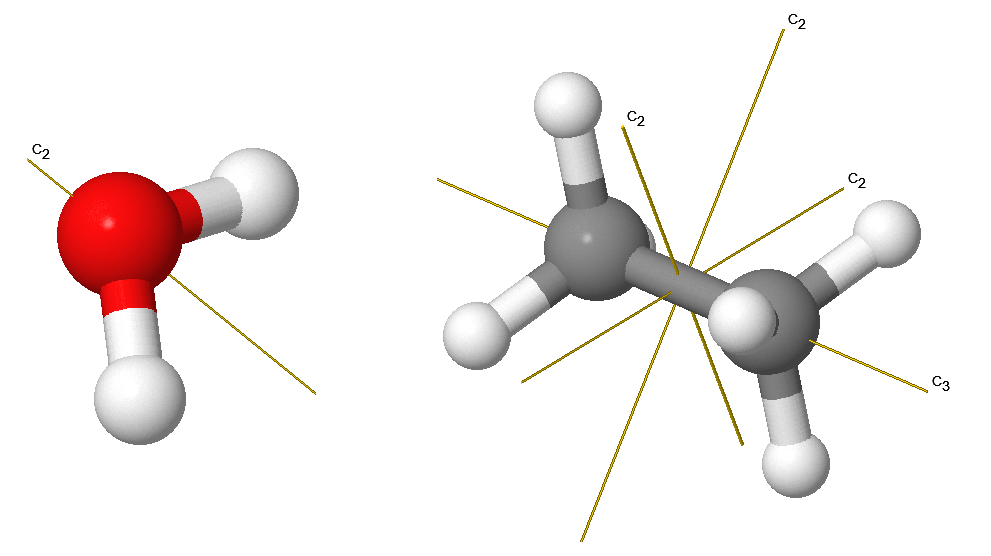
(CC BY-SA 4.0) : credit: Johnson et al. (using 'Symmetry')
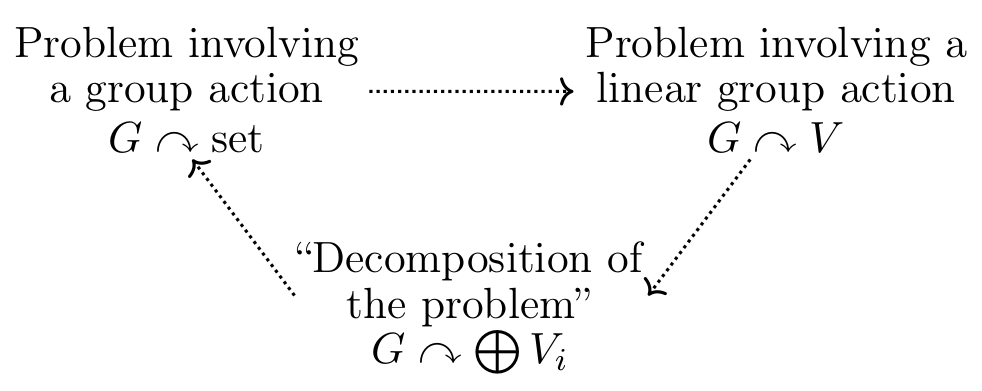
(CC BY-SA 4.0) : credit: Daniel Tubbenhauer
Class Meetings
- Winter 2025 (Jan13 → May3)
- MW 11:00-12:40PM
- Gries 325
- zoom link - passcode 631235
Instructor

Daniel Rowe
darowe{at}nmu{dot}edu
I'm an associate professor of mathematics in the Mathematics and Computer Science Department at Northern Michgan University. I've been a professor at NMU for nine years, and I am very passionate about the praxis of doing mathematics and teaching it. I grew up on a fishing camp in Northwestern Ontario, Canada.
Learning Outcomes
This graduate-level course offers an introduction to representation theory, a cornerstone of modern mathematics with deep connections to physics, geometry, and algebra. The course will cover the fundamental concept of a representations of group (finite or Lie-type), along with a review of linear algebraic notions such as subspaces, quotients, tensor products, Hom spaces, and symmetric and skew-symmetric tensors. Topics include the classification of simple and indecomposable representations, the representation theory of finite groups over the complex numbers, and the structural theory of simple Lie algebras over the complex numbers. Applications and connections to other areas of mathematics and physics will be highlighted throughout.
Need Math Help?
- Office Hours
- W 1-2, R 11-12, F 11-12
- JXJ 2228
- zoom link - passcode 809390
- read the relevant section(s) of our materials
- study all posted solutions
- re-watch the recorded lectures
Class Structure
- hybrid-flexible, in-person and over zoom
- strive for in-person attendance if possible!
- avoid becoming reliant on zoom and recordings!
- engagement is vital to learning mathematics (or anything)
- (50%) Homework
- (20%) Midterm Exam
- (30%) Final Exam
Grade Scale
A (92-100%)
A- (90-91%)
B+ (86-89%)
B (82-85%)
B- (80-81%)
C+ (76-79%)
C (72-75%)
C- (70-71%)
D+ (66-69%)
D (62-65%)
D- (60-61%)
F (≤ 59%)
Academic Honesty
In the spirit of academic honesty, credit for this section is due to Asher Auel, as this is an adapted form of their discussion of academic honesty in mathematics.
- Working with others on mathematics, and using electronic resources is both highly encouraged and fun. You may work with anyone (e.g. classmates, non-classmates, tutors, etc.) If this is done well, you'll learn more effectively and efficiently.
Here's the fundamental rule:
Work with anyone or anything to develop your own personal understanding of the ideas required to solve your homework problem, but always write-up the final draft by yourself and in your own words.
- Writing up the final draft is just as important as figuring out the problems on scratch paper with your friends, using the internet, ChatGPT, etc. If you work with people, or use electronic resources on a particular homework:
You must list your collaborators and electronic sources at the top of the very first page. This makes the process completely transparent and honest.
A Note About Copying Mathematics
Mathematical writing is idiosyncratic; if your assignments are copied, it is quite easy to tell. You will not learn by copying solutions from others, or from external sources such as internet forums (e.g. math.stackexchange) and generative AI (e.g. ChatGPT). Regarding internet forums, you are free to look at them and use any understanding you've gained from them. Be warned that internet forums often contain incorrect or circuitous solutions, misleading discussions, use of techniques outside of the course material, and other material that may be detrimental to your learning process. Even the time that it takes to repeatedly search for solutions and read through dozens of forum posts could be better spent learning the material on your own or composing a question to the instructor or classmate. Regarding generative AI (e.g. ChatGPT), you are free to experiment with asking questions, but be warned that these systems are currently still very bad at deductive reasoning, and that the output may contain a mix of correct, incorrect, and unverified statements. Ask them to prove something false, they will work hard to do so, often giving contradictory answers. Therefore, I would be very careful with using these tools as learning resources on your own.
Punishments
In this modern world of online resources, cheating, copying, copy-and-pasting, ChatGPT, etc.; it is now more important than ever that citizens develop the intelligence to use their own brain to solve problems. I want my classes to be a postive force in this regard, by promoting the principles of academic honesty, and punishing those who disrespect those principles. The first infraction will result in a 0% on the entire assigment and a stern warning. If there is a second infraction, I will pursue sanctions through the Dean of Students office.
Accessibility
If you have a need for disability-related accommodations or services, please inform the Coordinators of Disability Services in the Dean of Students Office at 2001 C. B. Hedgcock Building (227-1737 or disability@nmu.edu). Reasonable and effective accommodations and services will be provided to students if requests are made in a timely manner, with appropriate documentation, in accordance with federal, state, and University guidelines.
Reading Materials
- The main reference for the class will be my hand-written lecture notes.
- These notes will combine bits and pieces from the following sources:
- William Fulton and Joe Harris, Representation Theory - A First Course, Springer, 2004.
- Pavel Etingof, Oleg Golberg, et. al., Introduction to Representation Theory, American Mathematical Society, 2011.
- David Bishop, Group Theory and Chemistry, Dover, 1973.
- Jean-Pierre Serre, Linear Representations of Finite Groups, Springer, 1977.
- S. Sternberg, Group Theory and Physics, Cambridge University Press, 1994.
- as well as Richard Borcherds' YouTube lecture series
Homework + Exams
- master list of homework problems
- hw1: Q1-Q5 → sol (posted 2/4 @ 10:30am)
- hw2: Q6-Q8 → sol (posted 2/17 @ 8:00AM
- hw3: Q9-Q16 → sol (posted 3/19 @ 2:00PM)
- hw4: Q17-Q21 → sol (posted 4/23 @ 2:00PM)
- hw5: Q22-Q24 (due 4/30 @ 11:59PM) → sol
- midterm_exam → sol
- final_exam
Submitting Your Work
- please put a .pdf file inside our shared google folder
- the shared google folder will be titled w25_ma521_yourlastname
- I will share it with you within first two weeks of class
- please don't submit anything via email attachment
- name your files in an organized manner, for example: hw1_Jane_Smith.pdf
- always show your work and keep it organized
- indicate/circle/highlight your answers
- answer the questions in the correct order
Late Submissions
- for midterm and final: must be submitted on time
- all other submissions: no late penalty until
the solutions are posted, then -50%
Checking Your Grade
- you can check your grade anytime, look for
untitled spreadsheet in our shared folder,
it will appear after the first few assignments
have been graded
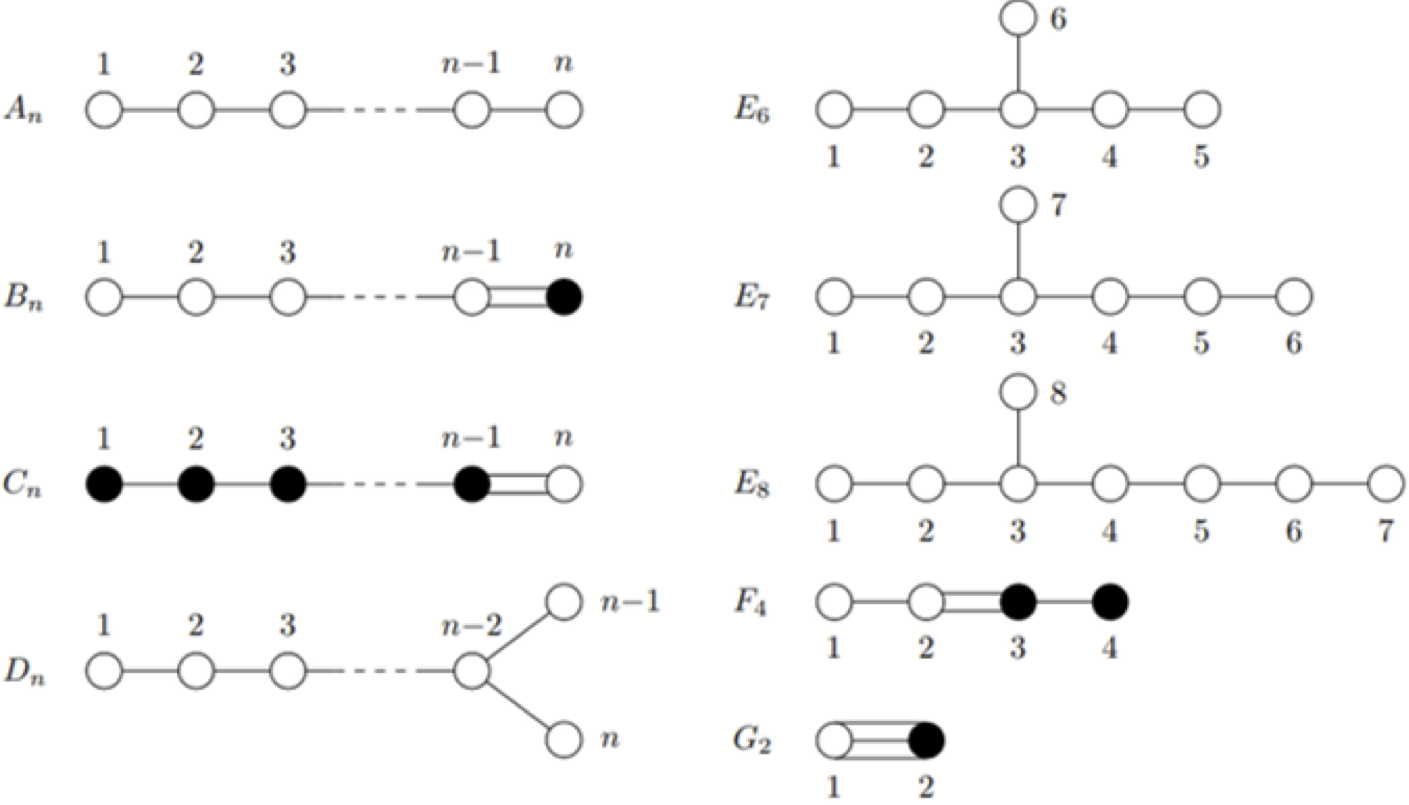
(CC BY-SA 4.0) : link
Schedule + Recordings
> colored text = clickable links
> late homework may be submitted anytime during the semester
> before the solutions are posted (-0%), otherwise (-50%)
wk1: jan13 → jan17
□ study the lectures
□ work on hw1
- 1/13
- introduction
- groups and examples
- 1/15
- matrix groups
- subgroup definition
- examples
- homomorphisms, isomorphisms
wk2: jan20 → jan24
□ study the lectures
□ work on hw1
- 1/22
- representations of a group
- examples
- simple/irreducible reps
- semi-simple reps
- chemistry analogy
wk3: jan27 → jan31
□ study the lectures
□ finish hw1
□ start hw2
- 1/27
- help with hw1
- another semi-simple representation
- a non semi-simple representation
- overview of the course
- direct products of groups
- 1/29
- direct products of groups
- conjugacy classes in groups
wk4: feb3 → feb7
□ study the lectures
□ keep working on hw2
- 2/3
- conjugacy classes in groups
- matrix conjugacy
- characteristic polynomials
- 2/5
- nilpotent operators
- matrix conjugacy classes
wk5: feb10 → feb14
□ study the lectures
□ start working on hw3
- 2/10
- a tour of general-
- -representation theory
- 2/12
- reps of finite groups
- characters
wk6: feb17 → feb21
□ study the lectures
□ start working on hw3
- 2/17
- discussion of hw2, hw3
- reps of finite groups
- character tables
wk7: feb24 → feb28
□ study the lectures
□ keep working on hw3
- 2/24
- more character tables
- groups, rings, fields
- 2/26
- no class - rowe travelling
wk8: mar10 → mar14
□ study the lectures
□ keep working on hw3
□ study for midterm exam next wed
- 3/12
- help with hw3
- abstract vector spaces
- examples
wk9: mar17 → mar21
□ study the lectures
□ finish hw3
□ midterm is this week
- 3/17
- midterm this week
- help with hw3
- 3/19
- quotients, hom spaces
- dual spaces, covectors
wk10: mar24 → mar28
□ study the lectures
□ start working on hw4
- 3/26
- tensor products
- symmetric and exterior powers
wk11: mar31 → apr4
□ study the lectures
□
- 4/2
- G-linear maps
- the regular representation
- G-invariant inner product
wk12: apr7 → apr11
□ study the lectures
□ finish up hw4
- 4/7
- character theory (proofs)
wk13: apr14 → apr18
□ study the lectures
□ start working on hw5
wk14: apr21 → apr25
□ study the lectures
□ finish up hw5
- 4/21
- tori and weight lattices
- root systems of Lie algebras
- classification of simple
- Lie algebras over C
- 4/23
- root systems of Lie algebras
- classification of simple
- Lie algebras over C
wk15: apr28 → may2 (FINAL EXAM WEEK)
□ take home final exam during exam week
□ due Thursday 5/1 @ 11:59PM



